Deflating Subspaces and Condition Numbers

The generalized Schur form depends on the order of the eigenvalues on the diagonal of (S,T) and this may optionally be chosen by the user. Suppose the user chooses that $(\alpha_1,\beta_1),\ldots,(\alpha_j,\beta_j), 1 \leq j \leq n$, appear in the upper left corner of (S,T). Then the first j columns of UQ and VZ span the left and right deflating subspaces of (A,B) corresponding to $(\alpha_1,\beta_1),\ldots, (\alpha_j,\beta_j)$.

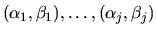The following routines perform this reordering and also compute condition numbers for eigenvalues, eigenvectors and deflating subspaces:

1.

xTGEXC will move an eigenvalue pair (or a pair of 2-by-2 blocks) on the diagonal of the generalized Schur form (S,T) from its original position to any other position. It may be used to choose the order in which eigenvalues appear in the generalized Schur form. The reordering is performed with orthogonal (unitary) transformation matrices. For more details see [70,73].

2.

xTGSYL solves the generalized Sylvester equations AR - LB = sC and DR - LE =sF for L and R, given A and B upper (quasi-)triangular and D and E upper triangular. It is also possible to solve a transposed system (conjugate transposed system in the complex case) A^T X + D^T Y = sC and -X B^T - Y E^T = sF for X and Y. The scaling factor s is set during the computations to avoid overflow. Optionally, xTGSYL computes a Frobenius norm-based estimate of the ``separation'' between the two matrix pairs (A,B) and (D,E). xTGSYL is used by the routines xTGSNA and xTGSEN, but it is also of independent interest. For more details see [71,74,75].

3.

xTGSNA computes condition numbers of the eigenvalues and/or left and right eigenvectors of a matrix pair (S,T) in generalized Schur form. These are the same as the condition numbers of the eigenvalues and eigenvectors of the original matrix pair (A,B), from which (S,T) is derived. The user may compute these condition numbers for all eigenvalues and associated eigenvectors, or for any selected subset. For more details see section 4.11 and [73].

4.

xTGSEN moves a selected subset of the eigenvalues of a matrix pair (S,T) in generalized Schur form to the upper left corner of (S,T), and optionally computes condition numbers of their average value and their associated pair of (left and right) deflating subspaces. These are the same as the condition numbers of the average eigenvalue and the deflating subspace pair of the original matrix pair (A,B), from which (S,T) is derived. For more details see section 4.11 and [73].

See Table 2.15 for a complete list of the routines, where, to save space, the word ``generalized'' is omitted.

Table 2.15: Computational routines for the generalized nonsymmetric eigenproblem

Type of matrix	Operation	Single precision		Double precision
and storage scheme		real	complex	real	complex
general	Hessenberg reduction	SGGHRD	CGGHRD	DGGHRD	ZGGHRD
	balancing	SGGBAL	CGGBAL	DGGBAL	ZGGBAL
	back transforming	SGGBAK	CGGBAK	DGGBAK	ZGGBAK
Hessenberg	Schur factorization	SHGEQZ	CHGEQZ	DHGEQZ	ZHGEQZ
(quasi)triangular	eigenvectors	STGEVC	CTGEVC	DTGEVC	ZTGEVC
	reordering	STGEXC	CTGEXC	DTGEXC	ZTGEXC
	Schur decomposition
	Sylvester equation	STGSYL	CTGSYL	DTGSYL	ZTGSYL
	condition numbers of	STGSNA	CTGSNA	DTGSNA	ZTGSNA
	eigenvalues/vectors
	condition numbers of	STGSEN	CTGSEN	DTGSEN	ZTGSEN
	eigenvalue cluster/
	deflating subspaces